Assignment #9

Pedal Triangles

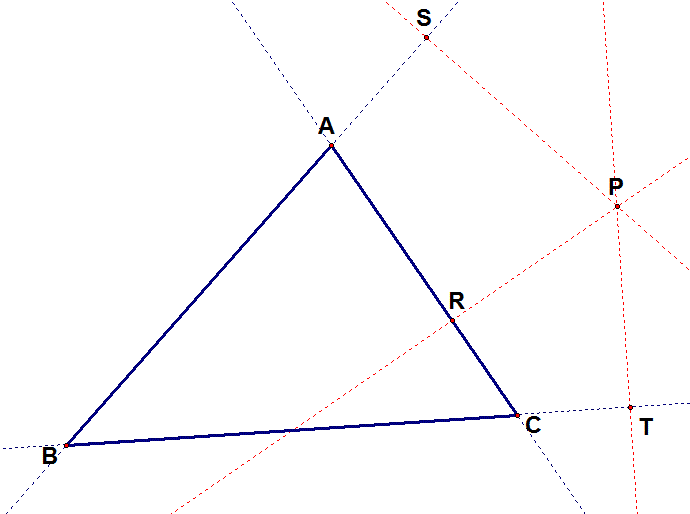
A pedal triangle is created from any triangle ABC and a point P in the same plane.

To construct the pedal triangle construct perpendicular lines to the sides of the triangle ABC (the sides may have to be extended) through point P.

The resulting intersections with the perpendicular lines and the triangle sides, points R, S, and T, are the vertices of the new pedal triangle RST for point P.

What happens when point P is the Orthocenter?

Conjecture: When point P is the orthocenter, the pedal triangle is also the orthic triangle.

Proof: The pedal triangle is created by constructing a perpendicular from point P to the sides of the triangle. The orthocenter is the common intersection of the altitudes of a triangle.

The orthic triangle is the triangle formed by the intersection of the altitude and the triangle sides.

When P is the orthocenter, the perpendicular lines projected from P lie o the altitude and, therefore, share the same intersection with the sides of the triangle. Since these common intersections of altitude and the triangle sides also form the orthic triangle, the conclusion can be made that when P is the orthocenter, the pedal triangle created is also the orthic triangle of triangle ABC.